Diff Eq's: Solutions to Problems in Section 4.4

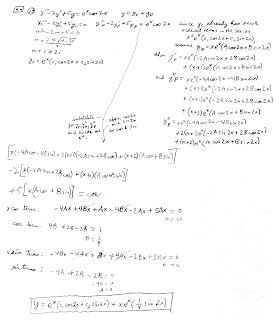
Here are solutions to problems #1-17 odd in Section 4.4 where we solve non-homogeneous linear DEs with constant coefficients, where the forcing functions are sums and products of polynomials, exponentials, and sine and cosine. The last few involve a great deal of bookkeeping to determine the coefficients.

Note that in #15, the forcing function has the same frequency as the natural frequency gotten from solving for the complementary solution. Therefore, you might have guessed the particular solution to be of the form x(A cos ωx + B sin ωx), but if you tried it, you found it didn't work. It would have worked if the forcing function weren't already multiplied by x. Since it is, we must instead propose a particular function with both sine and cosine multiplied by 2nd order polynomials with undetermined coefficients. (Actually, you could always err on the side of caution and propose higher order polynomial multiples. That would be a lot of useless busy-work, though, in cases where we can be sure of the order of the polynomial in the solution.)